.
The surface speed of the Earth's surface at the equator is roughly 1,040
mph, yet centrifugal force doesn't throw its oceans off into space.
Were I to spin a soccer ball so that the surface speed at its equator is 1,040
mph, all water on its surface will be thrown off except for maybe a small
mount at the poles of its axis.
FAQ: Why does water stay on the Earth's surface but not on the soccer ball's
surface?
REPLY: Gravity is directly proportional to mass.
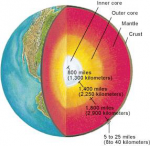
The Earth's mass, in pounds, is 1.31668×10 to the 25th power, which, as it
turns out, is sufficient mass to generate a gravity strong enough to prevent
centrifugal force from throwing water off its surface. Whereas the soccer
ball's mass is so insignificant in comparison that it cannot generate sufficient
gravity to hold water on its surface.
* A regulation soccer ball's diameter is roughly 27 inches, viz: .4444 feet,
which, when multiplied by pi yields a circumference 1.3963 feet. So we'd
have to roll the ball 3,781.5 times for its equator to measure out one mile.
Now multiply that by 1,040 and we get 3,932,679.2 rotations in 24 hours to
equal the Earth's surface speed @ one rotation in 24 hours. That's quite a
gear ratio.
_

2
1
1